Page 22 - 4234

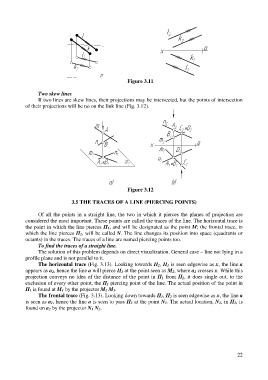
Figure 3.11

Two skew lines
If two lines are skew lines, their projections may be intersected, but the points of intersection
of their projections will be no on the link line (Fig. 3.12).

Figure 3.12

3.5 THE TRACES OF A LINE (PIERCING POINTS)

Of all the points in a straight line, the two in which it pierces the planes of projection are
considered the most important. These points are called the traces of the line. The horizontal trace is
the point in which the line pierces Π 1, and will be designated as the point M; the frontal trace, in
which the line pierces Π 2, will be called N. The line changes its position into space (quadrants or
octants) in the traces. The traces of a line are named piercing points too.
To find the traces of a straight line.
The solution of this problem depends on direct visualization. General case – line not lying in a
profile plane and is not parallel to it.
The horizontal trace (Fig. 3.13). Looking towards Π 2, Π 1 is seen edgewise as x, the line a
appears as a 2, hence the line a will pierce Π 1 at the point seen as M 2, where a 2 crosses x. While this
projection conveys no idea of the distance of the point in Π 1 from Π 2, it does single out, to the
exclusion of every other point, the Π 1 piercing point of the line. The actual position of the point in
Π 1 is found at M 1 by the projector M 2 M 1.
The frontal trace (Fig. 3.13). Looking down towards Π 1, Π 2 is seen edgewise as x, the line a
is seen as a 1, hence the line a is seen to pass Π 2 at the point N 1. The actual location, N 2, in Π 2, is
found on a 2 by the projector N 1 N 2.

22