Page 90 - 4749

- All the radii of the cross-section are straight, keep the length
and rotate at the same angle, i.e. each cross-section rotates around
the axis of the rod as a hard thin disk.
According to these results, the torsion causes shear
deformation of the material that is located between adjacent cross
sections.
For finding stresses in the cross-section of the rod in torsion we
study three aspects of this problem.

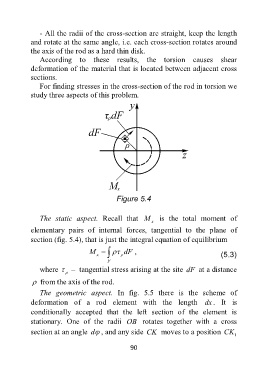
Figure 5.4

The static aspect. Recall that M is the total moment of
x
elementary pairs of internal forces, tangential to the plane of
section (fig. 5.4), that is just the integral equation of equilibrium

M    dF , (5.3)
x 
F
where  – tangential stress arising at the site dF at a distance

 from the axis of the rod.
The geometric aspect. In fig. 5.5 there is the scheme of
deformation of a rod element with the length dx . It is
conditionally accepted that the left section of the element is
stationary. One of the radii OB rotates together with a cross
section at an angle d , and any side CK moves to a position CK
1
90