Page 130 - 4624

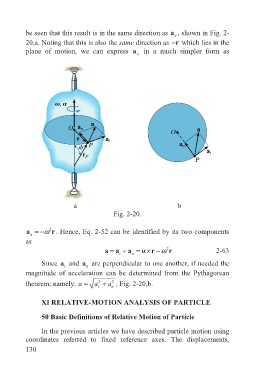
be seen that this result is in the same direction as a , shown in Fig. 2-
n
20,a. Noting that this is also the same direction as −r which lies in the
plane of motion, we can express a in a much simpler form as
n

a b
Fig. 2-20.

2
a n =− ω r. Hence, Eq. 2-52 can be identified by its two components
as
aa t + a n = ×− ω r 2-63
=
α r
2
Since a and a are perpendicular to one another, if needed the
n
t
magnitude of acceleration can be determined from the Pythagorean
2
2
theorem; namely, a = a + a , Fig. 2-20,b.
t
n
XI RELATIVE-MOTION ANALYSIS OF PARTICLE
50 Basic Definitions of Relative Motion of Particle
In the previous articles we have described particle motion using
coordinates referred to fixed reference axes. The displacements,
130