Page 128 - 4624

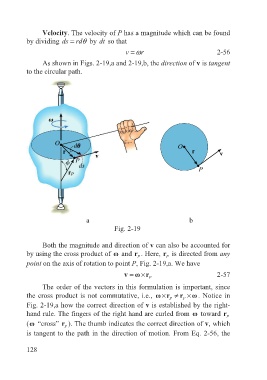
Velocity. The velocity of P has a magnitude which can be found
by dividing ds = rdθ by dt so that
v ω= r 2-56
As shown in Figs. 2-19,a and 2-19,b, the direction of v is tangent
to the circular path.

a b
Fig. 2-19

Both the magnitude and direction of v can also be accounted for
by using the cross product of ω and r . Here, r is directed from any
P
P
point on the axis of rotation to point P, Fig. 2-19,a. We have
×
v = ω r 2-57
P
The order of the vectors in this formulation is important, since
×
the cross product is not commutative, i.e., ω r P ≠ r P ×ω. Notice in
Fig. 2-19,a how the correct direction of v is established by the right-
hand rule. The fingers of the right hand are curled from ω toward r P
(ω “cross” r ). The thumb indicates the correct direction of v, which
P
is tangent to the path in the direction of motion. From Eq. 2-56, the

128