Page 54 - 4498

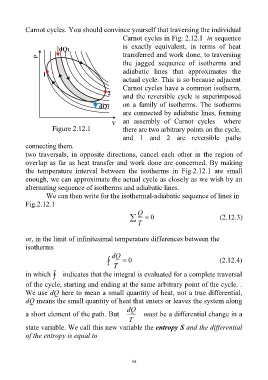
Carnot cycles. You should convince yourself that traversing the individual
Carnot cycles in Fig. 2.12.1 in sequence
is exactly equivalent, in terms of heat
transferred and work done, to traversing

the jagged sequence of isotherms and
adiabatic lines that approximates the
actual cycle. This is so because adjacent

Carnot cycles have a common isotherm,
and the reversible cycle is superimposed
on a family of isotherms. The isotherms
are connected by adiabatic lines, forming

an assembly of Carnot cycles where
Figure 2.12.1 there are two arbitrary points on the cycle,
and 1 and 2 are reversible paths

connecting them.
two traversals, in opposite directions, cancel each other in the region of
overlap as far as heat transfer and work done are concerned. By making

the temperature interval between the isotherms in Fig.2.12.1 are small
enough, we can approximate the actual cycle as closely as we wish by an
alternating sequence of isotherms and adiabatic lines.

We can then write for the isothermal-adiabatic sequence of lines in
Fig.2.12.1
Q
  0 (2.12.3)
T

or, in the limit of infinitesimal temperature differences between the

isotherms
dQ
  0 (2.12.4)
T
in which  indicates that the integral is evaluated for a complete traversal

of the cycle, starting and ending at the same arbitrary point of the cycle. .
We use dQ here to mean a small quantity of heat, not a true differential,

dQ means the small quantity of heat that enters or leaves the system along
dQ
a short element of the path. But must be a differential change in a
T
state variable. We call this new variable the entropy S and the differential
of the entropy is equal to

54