Page 18 - 4461

pressure, the gas must be allowed to expand; otherwise, its pressure will rise. As the gas
expands, it exerts a force on some piston and so does some work. In this case, the heat
supplied to the gas is used not only in raising the temperature of the gas but also in
doing some work. Thus a gas absorbs more heat at constant pressure than that it absorbs
at constant volume for the same rise of temperature:

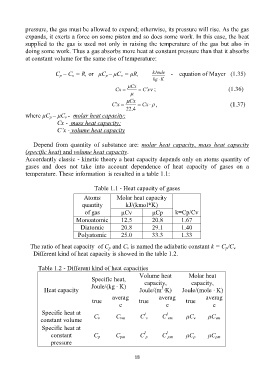
C p – C v = R, or μC p – μC v = μR, kJoule - equation of Mayer (1.35)
kg  K
Cx
Cx   C  x  ; (1.36)

Cx
xC   Cx   , (1.37)
22 4 .
where μC p – μC v - molar heat capacity;
Cx - mass heat capacity;
C’x - volume heat capacity

Depend from quantity of substance are: molar heat capacity, mass heat capacity
(specific heat) and volume heat capacity.

Accordantly classic - kinetic theory a heat capacity depends only on atoms quantity of
gases and does not take into account dependence of heat capacity of gases on a
temperature. These information is resulted in a table 1.1:

Table 1.1 - Heat capacity of gases
Atoms Molar heat capacity
quantity kJ/(kmol*K)
of gas μCv μCp k=Cp/Cv

Monoatomic 12.5 20.8 1.67

Diatomic 20.8 29.1 1.40

Polyatomic 25.0 33.3 1.33

The ratio of heat capacity of C p and C v is named the adiabatic constant k = C p/C v
Different kind of heat capacity is showed in the table 1.2.

Table 1.2 - Different kind of heat capacities
Volume heat Molar heat
Specific heat,
capacity, capacity,
Joule/(kg · K)
3
Heat capacity Joule/(m ·K) Joule/(mole · K)
averag averag averag
true true true
e e e
Specific heat at І І
C v C vm C v C vm μC v μC vm
constant volume
Specific heat at
І І
constant C p C pm C p C pm μC p μC pm
pressure

18