Page 7 - 4263

Determinants and their properties

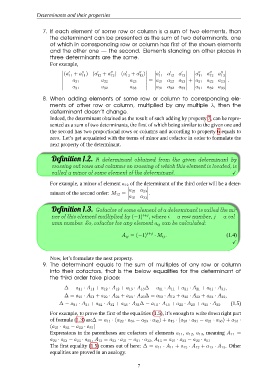
7. If each element of some row or column is a sum of two elements, than
the determinant can be presented as the sum of two determinants, one
of which in corresponding row or column has first of the shown elements
and the other one — the second. Elements standing on other places in
three determinants are the same.
For example,

a
a
(a + a ) (a + a ) (a + a ) ′ a ′ a ′ ′′ a ′′ a ′′
′′
′
′
′
′′
′′

11 11 12 12 13 13 11 12 13 11 12 13

= a 21 a 22 a 23 + a 21 a 22 a 23 .

a 21 a 22 a 23

a 31 a 32 a 33 a 31 a 32 a 33 a 31 a 32 a 33
8. When adding elements of some row or column to corresponding ele-
ments of other row or column, multiplied by any multiple λ, then the
determinant doesn’t change.
Indeed, the determinant obtained as the result of such adding by property 7, can be repre-
sented as a sum of two determinants, the first of which being similar to the given one and
the second has two proportional rows or columns and according to property 6 equals to
zero. Let’s get acquainted with the terms of minor and cofactor in order to formulate the
next property of the determinant.
Definition 1.2. A determinant obtained from the given determinant by
crossing out rows and columns on crossing of which this element is located, is
called a minor of some element of the determinant. ✓
For example, a minor of element a 12 of the determinant of the third order will be a deter-

a 21 a 23
minant of the second order: M 12 = .

a 31 a 33
Definition 1.3. Cofactor of some element of a determinant is called the mi-
nor of this element multiplied by (−1) i+j , where i — a row number, j — a col-
umn number. So, cofactor for any element a ij can be calculated:

A ij = (−1) i+j · M ij . (1.4)
✓

Now, let’s formulate the next property.
9. The determinant equals to the sum of multiples of any row or column
into their cofactors, that is the below equalities for the determinant of
the third order take place:

∆ = a 11 · A 11 + a 12 · A 12 + a 13 · A 13 ∆ = a 11 · A 11 + a 21 · A 21 + a 31 · A 31 ,
∆ = a 21 · A 21 + a 22 · A 22 + a 23 · A 23 ∆ = a 12 · A 12 + a 22 · A 22 + a 32 · A 32 ,

∆ = a 31 · A 31 + a 32 · A 32 + a 33 · A 33 ∆ = a 13 · A 13 + a 23 · A 23 + a 33 · A 33 . (1.5)
For example, to prove the first of the equalities (1.5), it’s enough to write down right part
of formula (1.3) as:∆ = a 11 · (a 22 · a 33 − a 23 · a 32 ) + a 12 · (a 23 · a 31 − a 21 · a 33 ) + a 13 ·
(a 21 · a 32 − a 22 · a 31 )
Expressions in the parentheses are cofactors of elements a 11 , a 12 , a 13 , meaning A 11 =
a 22 · a 33 − a 23 · a 32 , A 12 = a 23 · a 31 − a 21 · a 33 , A 13 = a 21 · a 32 − a 22 · a 31
The first equality (1.5) comes out of here: ∆ = a 11 · A 11 + a 12 · A 12 + a 13 · A 13 . Other
equalities are proved in an analogy.

7