Page 10 - 4811

Convergence
.

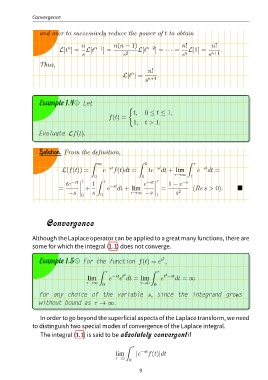
and over to successively reduce the power of t to obtain

n n(n − 1) n! n!
n
L[t ] = L[t n−1 ] = L[t n−2 ] = · · · = L[1] = .
s s 2 s n s n+1
Thus,
n!
n
L[t ] = .
s n+1
. . . .
.

Example 1.4, Let
{
t, 0 ≤ t ≤ 1,
f(t) =
1, t > 1.

Evaluate Lf(t).
. . . . .
.

Solution. From the definition,

∫ ∫ 1 ∫ τ
∞
L(f(t)) = e −st f(t)dt = te −st dt + lim e −st dt =
0 0 τ→∞ 1
1 ∫ 1 −st τ −s
−st
te 1 e 1 − e
= + e −st dt + lim = (Re s > 0).
−s s τ→∞ −s s 2
0 0 1
. . . .

Convergence

Although the Laplace operator can be applied to a great many functions, there are
.
some for which the integral (1.1) does not converge.

2
t
Example 1.5, For the function f(t) = e ,
τ 2 τ 2
∫ ∫
e dt = lim
lim e −st t e t −st dt = ∞
τ→∞ τ→0
0 0
for any choice of the variable s, since the integrand grows
without bound as τ → ∞.
. . . . .

In order to go beyond the superficial aspects of the Laplace transform, we need

to distinguish two special modes of convergence of the Laplace integral.
The integral (1.1) is said to be absolutely convergent if

∫
τ
lim |e −st f(t)|dt
τ→0
0
9