Page 50 - 4798

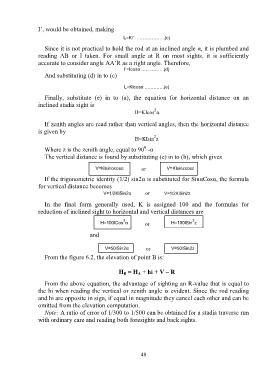
I’, would be obtained, making

L=KI’ ………………..(c)

Since it is not practical to hold the rod at an inclined angle α, it is plumbed and
reading AB or I taken. For small angle at R on most sights, it is sufficiently
accurate to consider angle AA’R as a right angle. Therefore,
I’=Icosα ……………(d)
And substituting (d) in to (c)

L=KIcosα ………….(e)

Finally, substitute (e) in to (a), the equation for horizontal distance on an
inclined stadia sight is
H=KIcos α
2

If zenith angles are read rather than vertical angles, then the horizontal distance
is given by
H=KIsin z
2
0
Where z is the zenith angle, equal to 90 -α
The vertical distance is found by substituting (e) in to (b), which gives

V=KIsinαcosα or V=KIsinzcosz

If the trigonometric identity (1/2) sin2α is substituted for SinαCosα, the formula
for vertical distance becomes

V=1/2KISin2α or V=1/2KISin2z

In the final form generally used, K is assigned 100 and the formulas for
reduction of inclined sight to horizontal and vertical distances are

2
2
H=100ICos α or H=100ISin z

and

V=50ISin2α or V=50ISin2z

From the figure 6.2, the elevation of point B is:

H B = H A + hi + V – R

From the above equation, the advantage of sighting an R-value that is equal to
the hi when reading the vertical or zenith angle is evident. Since the rod reading
and hi are opposite in sign, if equal in magnitude they cancel each other and can be
omitted from the elevation computation.
Note: A ratio of error of 1/300 to 1/500 can be obtained for a stadia traverse run
with ordinary care and reading both foresights and back sights.

48