Page 20 - 4560

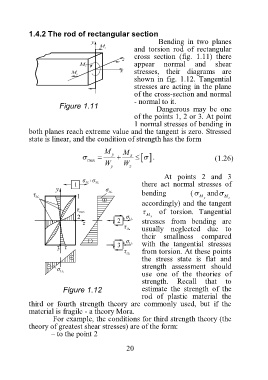
1.4.2 The rod of rectangular section
Bending in two planes
and torsion rod of rectangular
cross section (fig. 1.11) there
appear normal and shear
stresses, their diagrams are
shown in fig. 1.12. Tangential
stresses are acting in the plane
of the cross-section and normal
- normal to it.
Figure 1.11
Dangerous may be one
of the points 1, 2 or 3. At point

1 normal stresses of bending in
both planes reach extreme value and the tangent is zero. Stressed
state is linear, and the condition of strength has the form
M M

  y  z    . (1.26)
max
W W
y z
At points 2 and 3
there act normal stresses of
bending ( and
M y M z
accordingly) and the tangent
 of torsion. Tangential
M x
stresses from bending are
usually neglected due to
their smallness compared
with the tangential stresses
from torsion. At these points
the stress state is flat and
strength assessment should
use one of the theories of

strength. Recall that to
Figure 1.12 estimate the strength of the
rod of plastic material the
third or fourth strength theory are commonly used, but if the
material is fragile - a theory Mora.
For example, the conditions for third strength theory (the
theory of greatest shear stresses) are of the form:
– to the point 2

20